Not Squares?
There might be times when a model asks for a different paper dimension from the standard square that origami paper comes in. I probably have more models which start with non-square paper in fact. Here is a guide to some of the most common shapes you might require.
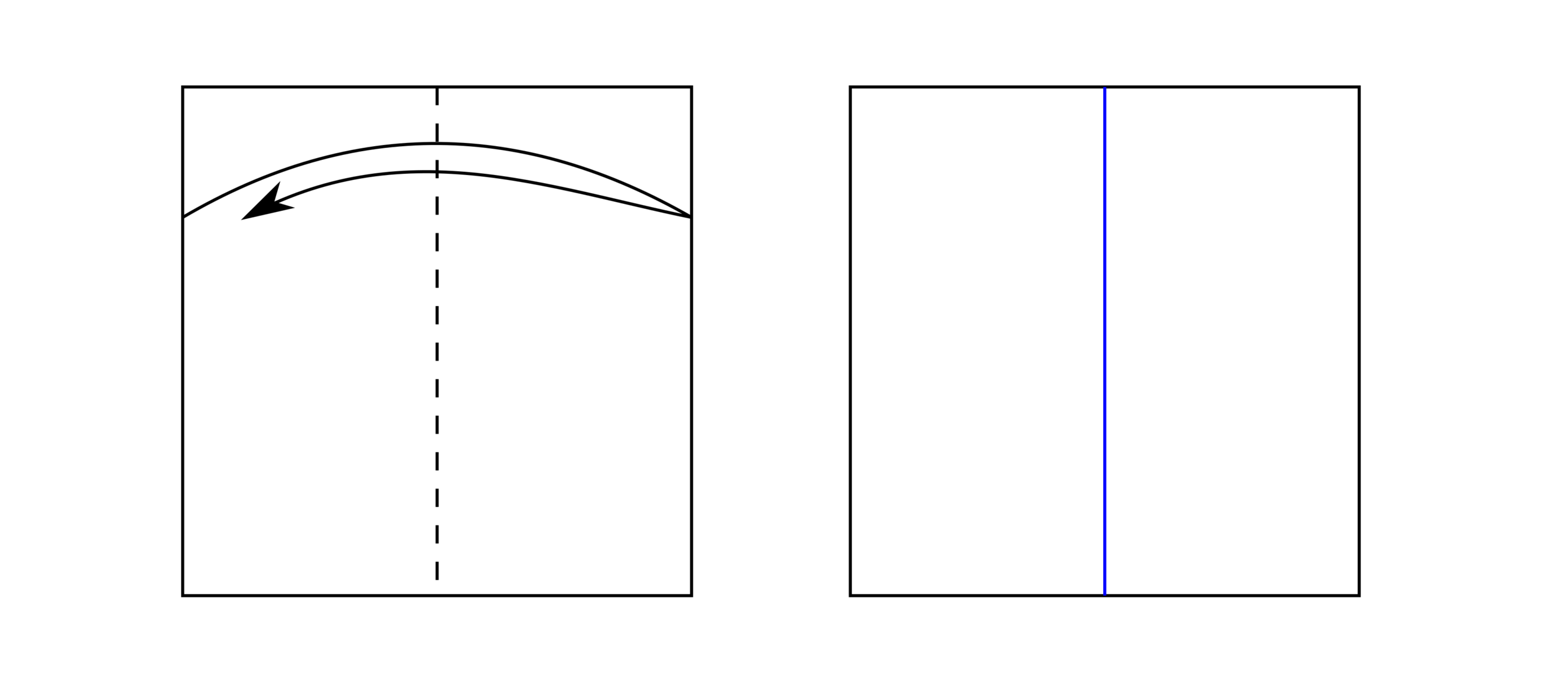
1:2 paper
Fold the paper in half and cut along this fold (the blue line). You will have two pieces of paper in 1:2 proportion.
1:3 paper
Pinch the paper midpoint. Form the lines from the top left to the mid bottom of the paper and the bottom right to top left and only crease the section where you expect them to intersect. Use this intersection point to find the thirds of the paper, fold the right side to meet this point and then fold the left side to meet the crease you just made.
1:4, 1:6, 2:3 paper
You can adapt other divisions by adding extra folds. In order (left to right, top to bottom): fold each section of the 1:2 paper in half again to get 1:4; Fold the sections of 1:3 in half again to get 1:6; Don't fold the second third measurement of 1:3 to get one 2:3 piece and one 1:3 piece; or if you want lots of 2:3 pieces fold the other midline of the 1:3 division.
1:√3 paper
Depending on the number of pieces of 1:√3 paper you want from each square, use one of these methods. In the second one the 1:√3 piece is the lower section.
2:√3, 4:√3, 1:2√3 paper
Adapt the first method from 1:√3. Either: only pinch the first fold and don't cut the middle line to achieve one piece of 2:√3 paper from a square; fold the middle line, but then fold the bottom edge to meet the horizontal crease (not the top of the paper) resulting in four pieces of 2:√3 paper; only pinch the first fold, but fold the bottom edge to the top crease to get two 4:√3 pieces of paper; fold the edges to the middle line to get four thin 1:2√3 pieces of paper.
5:√3 paper
Divide paper into thirds as shown above and then construct the additional folds and cut along the marked blue lines (the horizontal line is defined by the intersection point of two fold) to get 3 pieces of paper in 5:√3 proportions.
Hexagonal Paper
I recommend the diagram sequence found on go origami here (below the video). Maria's method is excellent and avoids most creases through the hexagon.
I hope this is helpful. If you know any other easy ways of folding useful dimensions that you think I should include feel free to contact me on my about page and I'll update this page.